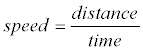
This excursion will focus on kinematics (the study of motion) problems where acceleration is zero. We will explore the meaning of the terms distance, displacement, speed, and velocity and develop the skills to calculate average velocity. You should have a paper printout of a worksheet that accompanies this web page in front of you.
The physlet above allows you to watch a ball moving horizontally for 10 seconds with a constant speed. At time t = 0 the ball is at the origin of the coordinate system. You may use the pause button to stop the animation and the step buttons to move through the animation frame by frame. You can click anywere on the grid and read out the x and y values (assume units are in meters) of the cursor location. Click Simulate! below to begin the animation.
To measure the speed of the ball, you should use the pause button to stop the ball soon after it starts and use the cursor to measure its position x1 while noting the value of time t1. Press play to continue the animation. Click pause once again to stop the animation with the ball further along in its path. Measure its position x2 while noting the value of time t2. The speed of the ball can then be evaluated with the following formula:
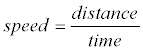
Note that speed is what physicists call a scalar quantity. It has magnitude (value), but no direction associated with it. Quantities with both a magnitude and a direction are called vectors. Distance is a scalar while displacement is a vector. The scalar speed is the magnitude of the vector velocity.
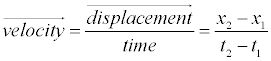
In this simulation both distance and displacement will have the same value at a given time, however they will be very different for more complicated motions. This occurs because distance is only concerned with how far the ball has traveled regardless of direction, while displacement indicates how far the ball has moved far away from the origin.
The physlet above allows you to watch a ball moving horizontally for 8 seconds with a constant velocity that you specify. Although the ball is still moving in 1-dimension, its velocity is a vector and the direction will be descibed by the sign. Simply select a velocity in the input field directly below and click Simulate! to begin the animation.
The physlet above allows you to watch a ball moving horizontally for 10 seconds with a constant speed. However, the velocity changes at t = 7 seconds. Thus, if we consider the motion over the entire 10 second interval, the average speed and average velocity are very different. This occurs because distance is only concerned with how far the ball has traveled regardless of direction, while displacement indicates how far the ball has moved far away from the origin. Output boxes associated with this physlet indicate the value of both the displacement and the distance. Click Simulate! to begin the animation.
The physlet above allows you to watch a ball moving horizontally for 10 seconds. For the first 5 seconds it moves with one velocity and for the second 5 seconds a second velocity. You can specify both velocities with the selection boxes below. Click Simulate! to begin the animation.